Bias and precision of methods for estimating the difference in restricted mean survival time from an individual patient data meta-analysis

Abstract

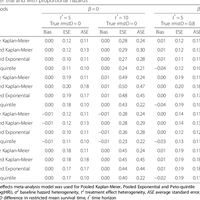
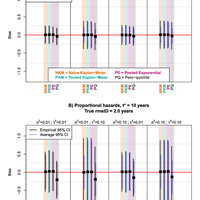
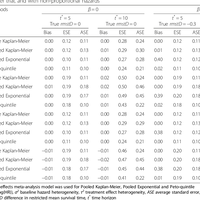
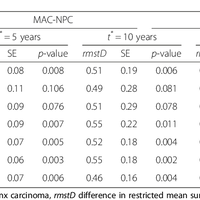
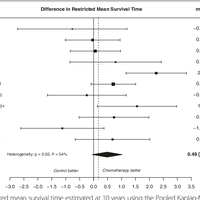
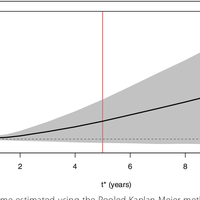
Background: The difference in restricted mean survival time (rmstD t∗), the area between two survival curves up to time horizon t∗, is often used in cost-effectiveness analyses to estimate the treatment effect in randomized controlled trials. A challenge in individual patient data (IPD) meta-analyses is to account for the trial effect. We aimed at comparing different methods to estimate the rmstD t∗ from an IPD meta-analysis. Methods: We compared four methods: the area between Kaplan-Meier curves (experimental vs. control arm) ignoring the trial effect (Naïve Kaplan-Meier); the area between Peto curves computed at quintiles of event times (Peto-quintile); the weighted average of the areas between either trial-specific Kaplan-Meier curves (Pooled Kaplan-Meier) or trial-specific exponential curves (Pooled Exponential). In a simulation study, we varied the between-trial heterogeneity for the baseline hazard and for the treatment effect (possibly correlated), the overall treatment effect, the time horizon t∗, the number of trials and of patients, the use of fixed or DerSimonian-Laird random effects model, and the proportionality of hazards. We compared the methods in terms of bias, empirical and average standard errors. We used IPD from the Meta-Analysis of Chemotherapy in Nasopharynx Carcinoma (MAC-NPC) and its updated version MAC-NPC2 for illustration that included respectively 1,975 and 5,028 patients in 11 and 23 comparisons. Results: The Naïve Kaplan-Meier method was unbiased, whereas the Pooled Exponential and, to a much lesser extent, the Pooled Kaplan-Meier methods showed a bias with non-proportional hazards. The Peto-quintile method underestimated the rmstD t∗, except with non-proportional hazards at t∗ = 5 years. In the presence of treatment effect heterogeneity, all methods except the Pooled Kaplan-Meier and the Pooled Exponential with DerSimonian-Laird random effects underestimated the standard error of the rmstD t∗. Overall, the Pooled Kaplan-Meier method with DerSimonian-Laird random effects formed the best compromise in terms of bias and variance. The rmstD (t∗ = 10 years ) estimated with the Pooled Kaplan-Meier method was 0.49 years (95 % CI: [-0.06;1.03], p = 0.08) when comparing radiotherapy plus chemotherapy vs. radiotherapy alone in the MAC-NPC and 0.59 years (95 % CI: [0.34;0.84], p < 0.0001) in the MAC-NPC2. Conclusions: We recommend the Pooled Kaplan-Meier method with DerSimonian-Laird random effects to estimate the difference in restricted mean survival time from an individual-patient data meta-analysis.