Skip to main content
Journal ArticleOPEN ACCESS

Quantum spin Hall phase in 2D trigonal lattice

Nature Communications (2016) 7
DOI: 10.1038/ncomms12746
48Citations
Citations of this article
53Readers
Mendeley users who have this article in their library.

This article is free to access.

Abstract

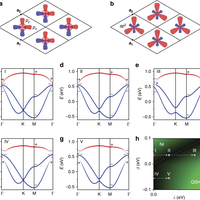
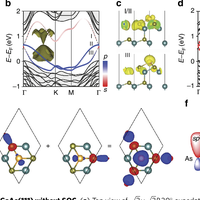
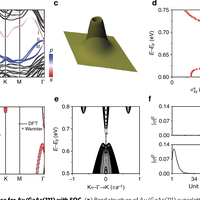
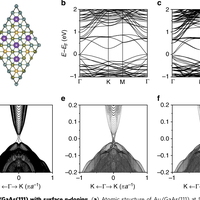
The quantum spin Hall (QSH) phase is an exotic phenomena in condensed-matter physics. Here we show that a minimal basis of three orbitals (s, p x, p y) is required to produce a QSH phase via nearest-neighbour hopping in a two-dimensional trigonal lattice. Tight-binding model analyses and calculations show that the QSH phase arises from a spin-orbit coupling (SOC)-induced s-p band inversion or p-p bandgap opening at Brillouin zone centre (" point), whose topological phase diagram is mapped out in the parameter space of orbital energy and SOC. Remarkably, based on first-principles calculations, this exact model of QSH phase is shown to be realizable in an experimental system of Au/GaAs(111) surface with an SOC gap of 1/473 meV, facilitating the possible room-temperature measurement. Our results will extend the search for substrate supported QSH materials to new lattice and orbital types.

Figures

  • Figure 1 | Minimal basis tight-binding model for QSH phase in a trigonal lattice. (a,b) Trigonal lattice with three orbitals (s, px, py) per lattice site and its equivalent three sp2 orbitals. a1 ¼ ð ffiffiffi 3 p =2; 1=2Þ and a2 ¼ ð ffiffiffi 3 p =2; 1=2Þ are lattice vectors. (c–e) The first-type band structures with parameter es¼0.83 eV, ep¼0 eV, tsss¼ –0.04 eV, tsps¼0.09 eV, tpps¼0.18 eV and tppp¼0.005 eV. l is 0, 0.03 and 0.08 eV for a,b and c, respectively. (f,g) The second-type band structures with es¼0.74 eV and l¼0, 0.03 eV for f,g respectively. The other parameters are the same to those in c–e. From c–g, the red and blue colours indicate the component of s and p orbitals, respectively, and the parities for each sub-band at time reversal invariant momenta are labelled with þ and signs. (h) Topological phase diagram in the parameter space of D¼ Es Ep (bandgap between s and p orbitals at G point without SOC) and l (SOC strength). The colour indicates the bandgap between top and middle band. The band structure parameters for c–g are marked by the dots with labels I–V in h. The dashed line is the boundary between normal insulator (NI) and QSH phase. The dashed arrows indicate the increasing SOC strength.
  • Figure 2 | Band, vortex and Berry curvature around the C point. (a) 3D band structure around the G point for bands I–III, illustrating the s–p band
  • Figure 3 | Band and orbital analysis for Au/GaAs(111) without SOC. (a) Top view of ffiffiffi 3 p ffiffiffi 3 p R 30 superlattice structure for Au grown on As-terminated GaAs(111) surface. (b) Band structure of Au/GaAs(111) superlattice without SOC. The inset is 3D plotting of I, II and III bands around G point. The red and blue colours indicate the component of s and p orbitals, respectively. (c) Charge density distribution of I, II and III bands at G point, showing the surface character. (d) Comparison between density functional theory (DFT) bands (solid lines) and MLWFs fitted bands (red dots). (e) Top view of three MLWFs fitted from the DFT bands and the overall orbital shape by adding them together. Red and blue colours denote positive and negative value, respectively. (f) Schematic view of the un-hybridized orbitals. One s orbital for Au and three sp3 orbitals for three As atoms, forming a tetrahedron structure. For clarity, only Au and the top two (one) bilayer GaAs atoms are plotted in c,e.
  • Figure 4 | Non-trivial topological phase for Au/GaAs(111) with SOC. (a) Band structure of Au/GaAs(111) superlattice with SOC. The red and blue colours indicate the component of s and p orbitals, respectively. (b) Zoom-in comparison between density functional theory (DFT) bands (solid lines) and MLWFs fitted bands (red dots) around the SOC bandgap. (c) Spin Berry curvature around the G point by setting the Fermi level within the energy window of SOC gap. (d) Spin Hall conductance as a function of Fermi level, showing quantized value within the energy window of SOC gap. (e) 1D ribbon band structure, showing gapless Dirac edge states within the energy window of SOC gap. (f) Real space distribution of the Dirac edge states at the energy marked by a blue dot in e. The two degenerate edge states are localized at opposite (left and right) edge of the ribbon.
  • Figure 5 | Intrinsic 2D QSH for Au/GaAs(111) with surface n-doping. (a) Atomic structure of Au/GaAs(111) at 5/12 coverage of K atoms. (b,c) Band structures without and with SOC. (d) 1D ribbon band structure, showing gapless Dirac edge states within the energy window of SOC gap. (e,f) 1D ribbon band structure projected onto edge unit cell, showing opposite (left and right) edge state, respectively. In the projected bands,

References Powered by Scopus

View more at Scopus

Cited by Powered by Scopus

View more at Scopus

Register to see more suggestions

Mendeley helps you to discover research relevant for your work.

Already have an account?

Cite

CITATION STYLE

APA

Wang, Z. F., Jin, K. H., & Liu, F. (2016). Quantum spin Hall phase in 2D trigonal lattice. Nature Communications, 7. https://doi.org/10.1038/ncomms12746

Readers over time

‘16‘17‘18‘19‘20‘21‘22‘23‘240481216

Readers' Seniority

Tooltip

PhD / Post grad / Masters / Doc 23

59%

Researcher 13

33%

Professor / Associate Prof. 3

8%

Readers' Discipline

Tooltip

Physics and Astronomy 31

74%

Materials Science 9

21%

Computer Science 1

2%

Chemistry 1

2%

Save time finding and organizing research with Mendeley

Sign up for free
0