Mathematical modeling of non-Fickian diffusional mass exchange of radioactive contaminants in geological disposal formations

Abstract

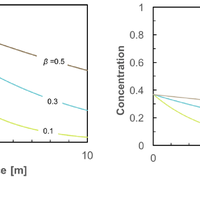
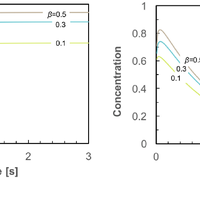
Deep geological repositories for nuclear wastes consist of both engineered and natural geologic barriers to isolate the radioactive material from the human environment. Inappropriate repositories of nuclear waste would cause severe contamination to nearby aquifers. In this complex environment, mass transport of radioactive contaminants displays anomalous behaviors and often produces power-law tails in breakthrough curves due to spatial heterogeneities in fractured rocks, velocity dispersion, adsorption, and decay of contaminants, which requires more sophisticated models beyond the typical advection-dispersion equation. In this paper, accounting for the mass exchange between a fracture and a porous matrix of complex geometry, the universal equation of mass transport within a fracture is derived. This equation represents the generalization of the previously used models and accounts for anomalous mass exchange between a fracture and porous blocks through the introduction of the integral term of convolution type and fractional derivatives. This equation can be applied for the variety of processes taking place in the complex fractured porous medium, including the transport of radioactive elements. The Laplace transform method was used to obtain the solution of the fractional diffusion equation with a time-dependent source of radioactive contaminant.