Noise Folding in Completely Perturbed Compressed Sensing

Abstract

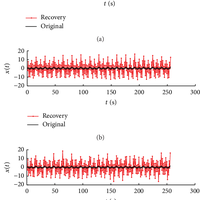
This paper first presents a new generally perturbed compressed sensing (CS) model y = (A + E) (x + u) + e, which incorporated a general nonzero perturbation E into sensing matrix A and a noise u into signal x simultaneously based on the standard CS model y = A x + e and is called noise folding in completely perturbed CS model. Our construction mainly will whiten the new proposed CS model and explore in restricted isometry property (R I P) and coherence of the new CS model under some conditions. Finally, we use OMP to give a numerical simulation which shows that our model is feasible although the recovered value of signal is not exact compared with original signal because of measurement noise e, signal noise u, and perturbation E involved.