Universal time dependence of the mean-square displacement in extremely rugged energy landscapes with equal minima

Abstract

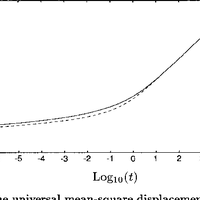
This paper presents a calculation of the time dependence of the mean-square displacement for symmetric random energy barrier hopping models at low temperatures, where the frequency dependence of the normalized diffusion constant D becomes universal, i.e., independent of the energy barrier probability distribution [J. C. Dyre, Phys. Rev. B 49, 11 709 (1994)]. The universal time dependence of the mean-square displacement is calculated from the effective medium approximation (EMA) universality equation, D lnD=s, where s is the dimensionless imaginary frequency, as well as for the approximation to the EMA universality equation Ds/ln(1+s). At long times the universal mean-square displacement is linear in time, corresponding to ordinary diffusion, whereas the mean-square displacement at short times t in dimensionless units varies as 2/ln(t-1). © 1995 The American Physical Society.