Feasibility of multiple micro-particle trapping—A simulation study

Abstract

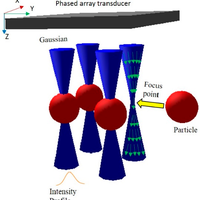
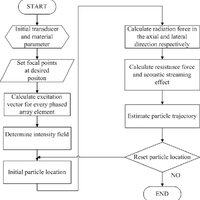
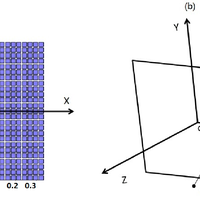
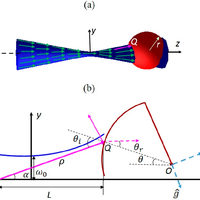
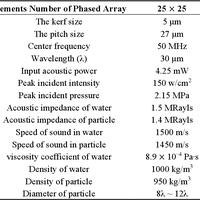
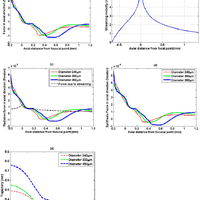
Both optical tweezers and acoustic tweezers have been demonstrated for trapping small particles in diverse biomedical applications. Compared to the optical tweezers, acoustic tweezers have deeper penetration, lower intensity, and are more useful in light opaque media. These advantages enable the potential utility of acoustic tweezers in biological science. Since the first demonstration of acoustic tweezers, various applications have required the trapping of not only one, but more particles simultaneously in both the axial and lateral direction. In this research, a method is proposed to create multiple trapping patterns, to prove the feasibility of trapping micro-particles. It has potential ability to electronically control the location and movement of the particles in real-time. A multiple-focus acoustic field can be generated by controlling the excitation of the transducer elements. The pressure and intensity of the field are obtained by modeling phased array transducer. Moreover, scattering force and gradient force at various positions are also evaluated to analyze their relative components to the effect of the acoustic tweezers. Besides, the axial and lateral radiation force and the trapping trajectory are computed based on ray acoustic approach. The results obtained demonstrate that the acoustic tweezers are capable of multiple trapping in both the axial and lateral directions.